INTRODUCTION
This blog aims to
define and discuss the key principles involved in executing an effective long jump.
A number of movement patterns will be identified and compared in order to
present one single optimal technique. Throughout the process of identifying the
movement patterns within long jump, many biomechanical concepts will
be presented and discussed in relation to
performance outcomes.
The International Association of
Athletics Federation (IAAF) (n.d.) stated that the goal of a long jumper is to
sprint along a runway and jump as far as possible into a sandpit from a
take-off board. This distance travelled, from the edge of the board to the
closest indentation in the sand to it, is then measured. Linthorne (2008)
builds upon this goal, stating this distance is greatly dependant on the
horizontal approach speed and the athletes’ ability to control, conserve and
dispense this speed over each phase. We have broken down the event of long
jump into four main phases: run up, take off, flight and landing. The biomechanical
principles specific to these phases will be discussed below.
MAJOR QUESTION
Run-up
There are various biomechanical principles involved in the initial run-up phase of long jump; it is a sprint requiring optimal speed, which is crucial as it affects the overall performance
(Bridgett & Linthorne, 2006; Linthorne, 2008). The athlete obtains optimal
biomechanical techniques by starting facing the pit, predominately with one
foot in front of the other, leaning forward and crouched down slightly
preparing to start a sprint (Linthorne, 2008) (displayed in Figure
1).
FIG. 1 See Pacey (2010) for full video
This starting position allows the
athlete to push off the ground with their back foot, causing them to move
forwards with maximal force; this can be contributed to Newton’s Laws of
Motion. Newton’s First Law is involved as the athlete remains at rest until a
net force larger than zero is applied (Blazevich, 2012), in this case, by the
athlete themselves. This force is influenced by inertia, and dependant on the
athlete’s mass; the force applied is assisted by having one foot in front of
the other, learning forward, and being slightly crouched down, instead of if
the athlete was standing upright. This also relates to Newton’s Second Law, as
the athlete needs to apply a large force to accelerate and push off the ground
(Blazevich, 2012). To maximise velocity, a large force production through the
legs and feet is required. The greater the athlete’s mass, or inertia, the
greater the force needed to change the state of motion and accelerate in a
constant horizontal running velocity (Blazevich, 2012). Therefore, the athlete
needs to accelerate from standing still to a high velocity sprint, applying a
force to overcome inertia and gravity to successfully move. The athlete will
accelerate faster as they apply a larger force to push off the ground. This
also creates an equal and opposite reaction according to Newton’s Third Law.
This means that as the athlete leans forwards, they push vertically downwards
into the ground with a force large enough to overcome inertia, exerting an
equal and opposite reaction (ground reaction force), accelerating the athlete
forwards when combined with some horizontal force as well (Figure 2) (Blazevich,
2012). These laws are not only relevant in the start position, but continued throughout
the whole run-up phase.
FIG. 2
A study by Bridgett and Linthorne
(2006) revealed when run-up and take-off speed increases, there is a positive
correlation with the distance jumped (shown in Figure 3). However, a later
study by Linthorne (2008) noted the athlete should accelerate quickly to
achieve near-maximal speed, aiming for 95-99% maximal effort. Research found
when athletes ran using 100% maximum effort it reduced foot placement accuracy,
which may result in fouled (unsuccessful) jumps. Top-speed should be reached
quickly as this improves average running speed resulting in greater performance
(Blazevich, 2012). The athlete decides on their run-up distance suited to
individual needs to ensure near-maximal speed is reached, generally 35-55
meters averaging in 16-24 strides (Linthorne, 2008). To calculate speed in
scalar quantity the change in distance is divided by the change in time of the
performance (Blazevich, 2012). The run-up is performed in a straight line
classifying it as rectilinear motion where the athlete moves with positive
displacement, distance, and acceleration (Blazevich, 2012).
FIG.3
FIG.4
Athletes need to swing their legs
rapidly when sprinting to increase speed. During running, the hips are the
principle aspect of torque production, and the centre of rotation when swinging
the legs (moment of inertia) (Blazevich, 2012). The ‘radius of gyration
describes the distribution of the mass relative to the centre of rotation’
(Blazevich, 2012, p. 74) and sprinters often reduce the weight in the calves to
increase muscle mass closer to the hips, reducing the moment of inertia, making
it easier to move (Blazevich, 2012). Torque is the force required to produce
angular velocity required for sprinting. We need this torque, or more simply,
power, to overcome inertia of the leg and ensure the athlete continues to move
with constant angular velocity (Blazevich, 2012). To increase torque, the legs
need to have a fast swing phase also reducing the loss of speed, and decreasing
moment of inertia (Blazevich, 2012). The leg also needs to be reasonably
extended during the swing phase and when landing on the ground. The recovery
phase also needs to occur quickly by flexing the leg up in front/under the body
(Figure 5), also aiming to increase angular velocity, while still reducing the
moment of inertia (Blazevich, 2012). The quicker the arm swings in a forward
motion while being tucked into the body, the more angular momentum it retains
(Blazevich, 2012) which reduces the moment of inertia and radius of gyration
further. Conservation of momentum is largely due to the arm swings, as the
opposite arm and leg swings at the same time (displayed in Figure 5); the arms
counteract the legs’ rotational momentum by producing an equal and opposite
angular momentum (Blazevich, 2012). The backward swing phase needs to be
vigorous to accelerate the body forward and upward as this increases running
speed. Whereas, it is extremely important that the recovery arm moves forward
slower than the backward/downward arm swing, otherwise it will push the body
backwards, resulting in losing speed (Blazevich, 2012). Therefore, force
production needs to be controlled, with correct equal and opposite reactions
for optimal technique and performance.
FIG. 5
Take-off
Although long jump performance is
primarily determined by the athlete's ability to reach a fast horizontal
velocity at the end of the run-up, the athlete must also use an appropriate
take-off technique to best conserve this momentum. The distance an athlete
jumps is largely determined by the flight distance (Hay, 1993) and this is
determined by the height, speed, and angle of projection of the centre of mass
at take-off. The speed and angle of projection are determined by the
combination of horizontal and vertical velocity. The horizontal velocity is
developed through the run-up, where the athlete is usually close to maximum
speed at the take-off board; whereas, vertical velocity is generated during
contact with the board (Lees & Fowler, 1994; Linthorne, 2008). If the
athlete is able to generate near maximum speed close to the board, the problem
long jumpers face is how to best generate vertical velocity from the board.
Adjustments can be made by the athlete when approaching the board to enable
vertical velocity to be generated.
The first step to improve a long
jumper’s take off technique is to analyse the last two strides taken in the
approach phase. The second to last stride before take-off should be longer than
the last, where the athlete lowers their body and centre of gravity (shown with
the slight dip of the hip in Figure 6). Continuing this, the last stride is
shorter while maintaining the lowered centre of gravity. These strides are significant,
and need to be near-maximal speed with horizontal velocity which has greater
kinetic energy utilised and transferred over to the vertical velocity in the
take-off and flight (Blazevich, 2012; Linthorne, 2008). Long jumpers need to place
their take-off foot well ahead of their centre of mass at touch down to produce
the necessary low position at the start of the take-off (Hay, 1993; Lees &
Fowler, 1993; Linthorne, 2008; Jaitner, Mendoza & Schöllhorn, 2001). This
is shown in Figure 7 where the centre of mass is represented by the yellow
line. The athlete’s take-off leg is the furthest point from the rest of the
body. As the athlete extends their leg longer than usual, the centre of mass is
not only horizontally changed, but vertically changed as well.
FIG.7
The lowering of the centre of mass
minimises the downward vertical velocity so as to maximize the effect of the
vertical impulse and increase the vertical distance over which the centre of
mass is worked. The large touchdown distance has been explained as enabling an
increase in the time period during which vertical impulse can be generated,
increasing the range of movement through which the hip extensor muscles may
work, and placing the leg in a position to enable it to be stretched and store
elastic energy which recoils upon release. In
this position the body can pivot over the leg to gain vertical velocity.
Unlike the projectile athletic
field events of javelin, shot put and discus, the long jump requires an angle
of release lower than 45° (Blazevich, 2012, Lees & Fowler, 1993; Linthorne
2008). The notion that the optimum take-off angle is 45° is based on the
assumption that the take-off velocity is constant for all moments of the
take-off phase. If the athlete
were to exhibit a take-off angle closer to 45° we would notice a significant
drop in velocity in order to manoeuvre the body segments in a way to allow
this. Additionally, a 45° angle of release assumes the vertical and horizontal
velocities are the same (Linthorne, 2008), which in the long jump is not
possible. Linthorne (2008) found long jumpers can reach significantly higher
horizontal velocities (8-10 m/s) compared to vertical velocities (3-4 m/s). Linthorne (2008) found when long jumpers produced near-maximal effort in
the run-up phase, the athletes' take-off angles from the board were less than
25 ̊. It was suggested
that to produce a jump with a larger take-off angle, athletes would need to slow
the run-up so take-off velocity can be reduced. As we discussed above, the greatest determinant to reach distance in the long jump is attaining a high velocity. Therefore, to slow the run up phase simply to increase take-off angle, would not improve performance. Instead, jumping at a lesser angle should see the athlete jump further. We analysed the jumps of three
of the world’s top long jumpers with specific focus to the take-off angle, and our
findings substantiate those of Linthorne. Our calculations show the long
jumpers all leaving the take-off board at angles less than 25°. We suggest coaches and teachers of the long jump make use of technology; whether it be mobile phone video, Ipad or Tablet Applications or specialised video equipment, to help break down the angle of take-off when training athletes. This can help improve the overall distance jumped.
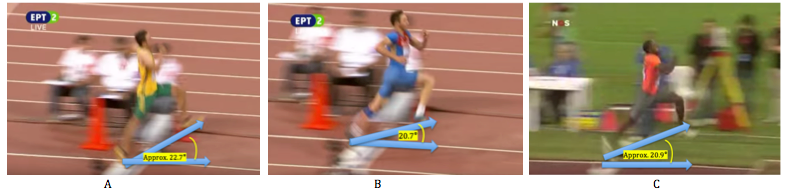
FIG.
8 Shows the take-off angle of three internationally acclaimed long jumpers: (A)
Fabrice Lapiere from Australia jumped a distance of 8.1 at the Beijing 2015
World Championship with a take-off angle of 22.7°, (B) Aleksandr Menkov also
competing at Beijing jumped 8.02 with a take-off angle of 20.7° and (C) Dwight
Phillips from USA jumped 8.6m at the Helsinki World Championship with an angle
of approximately 20.9°.
Another important factor to
consider when maximising energy conservation in the take-off phase is the
surface material of the board and the shoes worn by the athlete. In the same
way that we lose energy when we become fatigued, we lose energy when it gets
absorbed into other objects. In other words, a long jumper should try to
minimise energy lost when landing on, and pushing off the wooden board. The
coefficient of restitution tells us when an object collides with another object
or surface, a portion of the energy that the moving object has will be lost and
absorbed by the colliding object (Blazevich, 2012). To counteract the energy
lost during take-off, long jumpers use shoes specifically designed for their
event that have spikes under the ball of the foot and stiff soles (Linthorne,
2008). Stiff soles are biochemically advantageous for long jumpers to help
increase the coefficient of restitution as the ground is compact and the shoes
are also stiff, the majority of the energy from foot-ground contact will be
redistributed back to the athlete to increase distance jumped.
Flight
This phase aims to gain as much
time airborne, where the athlete’s body and centre of mass travels the furthest
horizontal distance (Bartlett, 2007). There are different flight techniques
athletes are shown to generally adopt; there is the sail, hang, and hitch-kick,
all shown in Figure 9. The hang technique is when the athlete looks like they
‘hang’ in the air bringing their limbs together almost extending them to slow
forward rotation. The sail is where the athlete almost floats through the air
in the same position from take-off for as long as possible. Finally, the
hitch-kick is where the athlete basically continues to run through the air. All
techniques basically end the same, where the athlete moves their body almost to
a pike position preparing for landing. These techniques often depend on the
run-up and take-off phases (Stefanović, 2015).
FIG.9
It is shown that an increased
run-up and take-off speed increases the distance jumped in the flight phase
(Bridgett & Linthorne, 2006). The athlete is ultimately a projectile as
they propel themselves into the air and end contact with the ground (in free
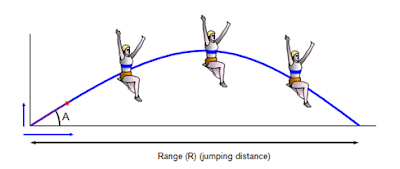
fall), occurring in a curvilinear motion (Bartlett, 2007). The athlete aims to
increase their trajectory more so their horizontal distance (range), than their
vertical motion to accelerate and jump through the air aiming for the furthest
distance (shown in Figure 10). This involves projectile motion where the
athlete is affected predominately by gravity, but also air resistance
(Blazevich, 2012; Linthorne, 2008). Newton’s First Law is involved, as well as
the Law of Gravitation as the athlete moves vertically they are pulled by
gravity affecting their state of motion (Bartlett, 2007; Blazevich, 2012). The
small amounts of air resistance present can be reduced by bringing all limbs
forward in a tuck/pike position to create a streamline effect. However,
according to Bartlett (2007) this position is also shown to negatively affect
performance by producing more forward rotation of the body, negatively
impacting the landing distance. Projectile velocity determines the amount of
time in the air and should continue the speed applied in the previous phases, which
is why a faster speed is preferable (Blazevich, 2012). Even though flight
technique is significant, the run-up and take-off speed ultimately determine
the distance. This projection speed also influences the range travelled,
multiplying the horizontal velocity by the flight time (Blazevich, 2012).
Regardless of air resistance, it is evident that gravity accelerates objects at
a rate of 9.81 ms-2 towards the ground (Blazevich, 2012). This means that all
objects accelerate the same, so athletes need to try increase their horizontal
range and trajectory within the time it takes to reach the ground. The
projection angle from take-off also impacts the distance travelled, which is
explained earlier in the ‘take-off’ phase.
FIG.10
Once the take-off is performed, the
athlete needs to control the forward rotation produced to result in a further
distance travelled (Linthorne, 2008). Generally, the amount of angular
momentum, along with the available time in the air before landing, determines
the flight technique. Often, coaches tell a novice that if they have higher
angular momentum then the hitch-kick is preferred, whereas, if they have lower
angular momentum, then often the hang technique is generally required
(Linthorne, 2008). Whereas, predominately, professional athletes are shown to
perform the hang or hitch-kick technique in the flight phase (Linthorne, 2008).
Both of these techniques allows the forward rotation to best occur and be
controlled, and also enables good landing positions. Angular momentum occurs
during flight phase; the hang technique attempts to reduce the forward
rotation, but also involves a large moment of inertia as the body is
predominately extended for majority of the flight, which reduces forward
velocity. Altering limb movement, either closer or further from the centre of
mass, changes the athlete's moment of inertia. Whereas, the continuous forward
rotation of the arms and legs along with straight posture, in the hitch-kick
technique conserves and transfers angular momentum from previous phases and
counteracts the forward rotation of the body (Bartlett, 2007). The rotation of
the arms and legs produces an equal and opposite backward angular momentum
which according to biomechanic principles will propel the athlete forwards.
The last section of the flight
prepares the athlete for landing, where they need to extend themselves the
furthest possible distance while still in the air to increase the horizontal
line distance travelled. This is done by ensuring the legs and arms are out in
front of the athlete (almost in a sitting position) while still leaning forward
to ensure they fall in front of where their legs touched the ground, to create
the furthest distance and not fall backwards which could cost them the
competition (Linthorne, 2008; Stefanović, 2015).
Landing
We know the goal of long jump, more
specifically, the landing, is to create the furthest distance between the take
off line and the closest mark made in the sandpit by the athlete. It was long
thought the optimum landing position for a long jump athlete is one with the
trunk nearly erect, legs below the horizontal line, and the arms behind the
body (Dyson, 1962; Schmolinsky, 1983). Recent developments in biomechanics
suggest otherwise. The findings from Mendoza (1989), Hay (1993), and Linthorne
(2008) found the optimum landing position is one with the hips fully flexed and
the trunk well forward over the legs (Figure 11).
FIG. 11
For the optimal landing technique,
we propose the athletes’ heels should make connection with the ground first. As
the flight phase ends, the athlete must prepare for landing by lifting their
legs up and extending them in front of the torso. As seen in the diagram above
(Figure 11), the heels should land just before the body’s projected flight path
(as represented by the dotted line). As the feet make contact with the sand,
the athlete can then press their heels downwards and contract the
hamstrings, which will in turn, cause the hips to rise. This sequential
movement pattern allows the conserved forward momentum from the previous three
phases, to carry the body past the landing position for the longest possible
jump.
THE ANSWER
From examining the stages of the
long jump and the biomechanical principles involved, there are some clear
answers into what an athlete can do to increase their distance jumped. Firstly,
to maximise distance, it is important to reach near-maximal speed when
performing the run up. As discussed earlier, specific technical cues can be
followed to increase leg and arm swing power, which result in a faster run-up.
An athlete can improve the speed of their sprint by conditioning their body to
increase the mass of muscles at the hips and decrease the mass of their calves.
There are also performance principles that underpin the take-off, flight and
landing phases of long jump. These include leaving the board at an optimum
angle for each individual athlete, providing it is well under 45°, a strong arm
swing for momentum and the ability to manipulate one’s centre of mass to
further horizontal distance upon landing. Improving on these personal
characteristics as well as the technique of the skill itself will produce a
more accurate, powerful, and overall effective distance jumped.
Dissimilar to other sports such as
basketball or tennis, an optimal technique is a fundamental aspect to executing
a successful long jump. Sports such as netball and tennis can have ideal
techniques, however with some players, their performance falls outside the
“optimal technique”. Long jump however does have a preferred technique that is
a combination of a number of factors such as posture, centre of gravity, ground
contact time, and manipulating free-swinging limbs.
HOW ELSE CAN WE USE THIS INFORMATION?
The biomechanical principles that
have been discussed throughout this blog are not limited to the long jump event
and are transferable into countless other sports and activities. Understanding
how to execute a skill with the optimal biomechanical technique is a
fundamental aspect to ensure peak performance.
In the run-up phase we noted the
optimal skill cue is to reach near-maximal velocity through a variety of
movement patterns, such as foot-ground contact, angular velocity of limbs and
torque. We can use this knowledge in sports where acceleration and velocity are
required, typically the faster the athlete, the more successful the
performance. Individual sports such as sprinting, hurdles and swimming are
great examples where an athlete needs to move as fast as possible to win. Similar,
are team tactical sports such as netball, soccer, basketball and AFL where
players need to move around the court or field to maximise performance.
Knowing the optimum angle of
release is helpful for sports with a jump take off, or releasing projectiles.
Being able to maximise the horizontal distance traveled, with reference to the
flight trajectory path, is crucial to sports such as discus, javelin and
cricket batting. While the execution of these activities is not identical to
long jump, the principles of manipulating angles of release are helpful to
perform successfully.
Being able to manipulate one’s mass
(body) can be helpful to develop strategies when participating in sports where
the athlete needs to evade an object or opponent; high jump is a good example
of this. The high jumper must try and manoeuvre their body segments when
passing over the bar to maximise the height between themselves and the bar.
Other sporting examples of centre of mass manipulation include, but are not
limited to, gymnastics, diving and basketball.
Overall, the aspects of long jump
can be linked quite effectively with triple jump. Similar to long jump, in
triple jump the athlete is required to reach high velocity in the run-up,
conserve momentum through take-off and flight and land with as much horizontal
distance into the sandpit as possible.
REFERENCES
Bartlett, R. (2007). Introduction to sports biomechanics: Analysing human
movement patterns (2 ed.). Routledge. London and New York.
Blazevich, A. J. (2012). Sports biomechanics: The basics: Optimising
human performance (2e). Bloomsbury. London: A&C Black
Publishers.
Bridgett, L. A., & Linthorne, N. P. (2006). Changes in long jump
take-off technique with increasing run-up speed. Journal of sports
sciences, 24(8), 889-897.
Hay, J. G. (1993). Citius, altius, longius (faster, higher, longer): the
biomechanics of jumping for distance. Journal of biomechanics, 26,
7-21.
International Association of Athletics Federations (IAAF). (n.d.) Long
Jump. Retrieved from http://www.iaaf.org/disciplines/jumps/long-jump
Jaitner, T., Mendoza, L., & Schöllhorn, W. (2001). Analysis of the
long jump technique in the transition from approach to takeoff based on time-continuous
kinematic data. European Journal of Sport Science, 1(5), 1-12.
Lees, A., Graham-Smith, P., & Fowler, N. (1994). A biomechanical
analysis of the last stride, touchdown, and takeoff characteristics of the
men's long jump. Journal of applied Biomechanics, 10,
61-61.
Linthorne, N. P. (2008). Biomechanics of the long jump. Handbook
of biomechanics and human movement science, 340-354.
Pacey, J. (2010). How to improve your long jump technique. YouTube:
Teach PE. Retrieved from https://www.youtube.com/watch?v=5v9p5jBN_Hg
Stefanović, R. (2015). Choice of
Exercises for Results in a Long Jump Performed with Different Techniques. Activities
in Physical Education and Sport. 5(2), 167-170.







No comments:
Post a Comment
Note: only a member of this blog may post a comment.